
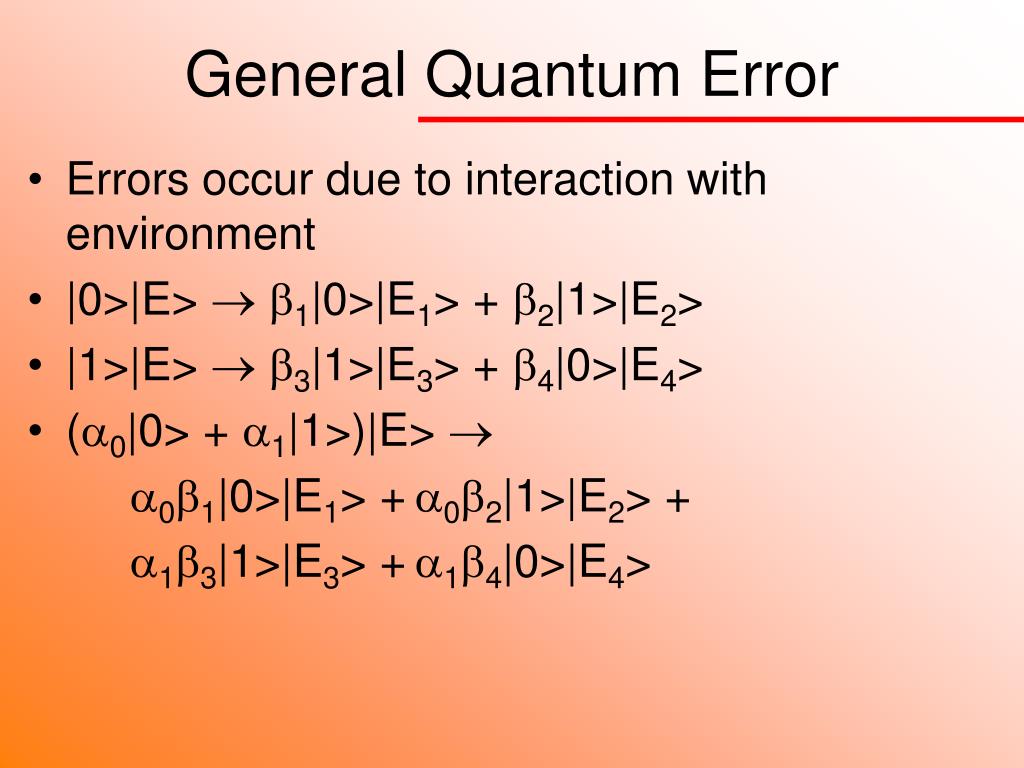
Similar to classical error correction, QEC codes do not always correctly decode logical qubits, but their use reduces the effect of noise.Ĭopying quantum information is not possible due to the no-cloning theorem. In this example, the logical information was a single bit in the one state, the physical information are the three copied bits, and determining what logical state is encoded in the physical state is called decoding. It is possible that a double-bit error occurs and the transmitted message is equal to three zeros, but this outcome is less likely than the above outcome. Assuming that noisy errors are independent and occur with some sufficiently low probability p, it is most likely that the error is a single-bit error and the transmitted message is three ones. Suppose further that a noisy error corrupts the three-bit state so that one of the copied bits is equal to zero but the other two are equal to one. suppose we copy a bit in the one state three times. The idea is to store the information multiple times, and-if these copies are later found to disagree-take a majority vote e.g. The simplest albeit inefficient approach is the repetition code. Ĭlassical error correction employs redundancy. This would allow algorithms of greater circuit depth. Quantum error correction is theorised as essential to achieve fault tolerant quantum computing that can reduce the effects of noise on stored quantum information, faulty quantum gates, faulty quantum preparation, and faulty measurements. Brun, " Optimal entanglement formulas for entanglement-assisted quantum coding", Physical Review A, June 2008.Quantum error correction ( QEC) is used in quantum computing to protect quantum information from errors due to decoherence and other quantum noise. Sarvepalli, " Nonbinary Stabilizer Codes Over Finite Fields, IEEE Trans. Sloane, " Quantum Error Correction via Codes over GF(4)", IEEE Trans.

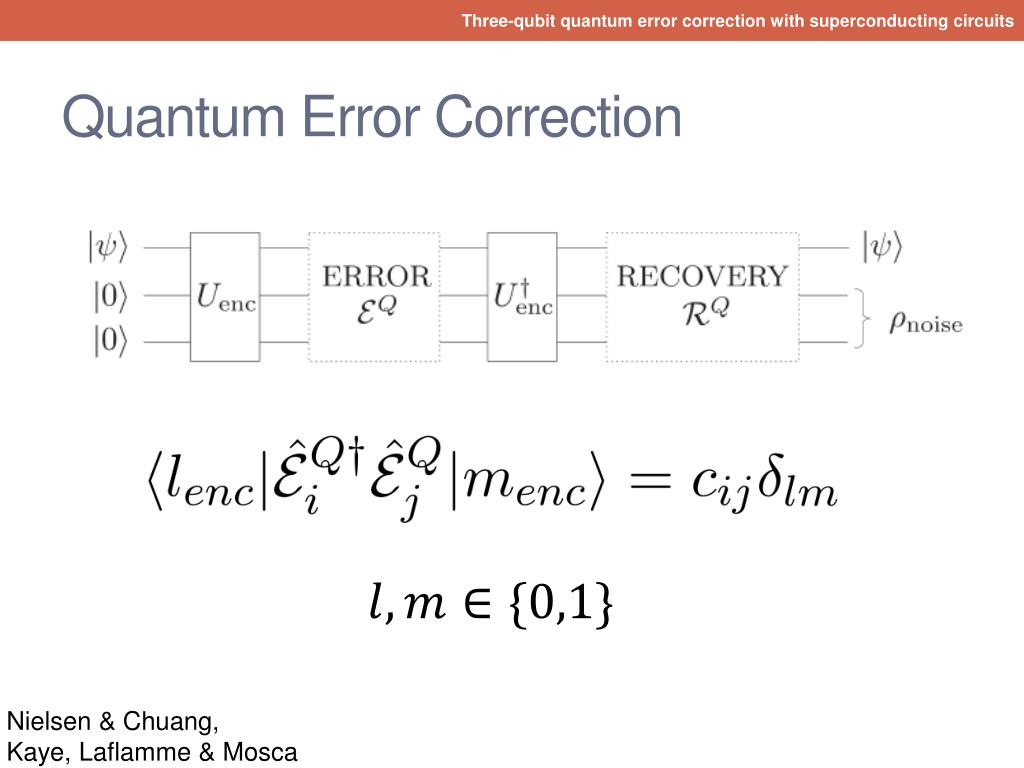
Chuang, Quantum Computation and Quantum Information,Ĭambridge University Press, 2010 (10th Anniversary Edition) Entanglement-assisted quantum error-correcting codes.Bounds: quantum Hamming bound, quantum Singleton bound, quantum Gilbert-Varshamov bound.Constructions: Shor's code, CSS codes, stabilizer codes, topological codes (surface codes, color codes), quantum LDPC codes.Quantum error-correction: quantum codes the Knill-Laflamme conditions Pauli error basis discretization of quantum errors.Quantum noise models: bit flips and phase flips, depolarizing channel, amplitude damping, phase damping.Basics of quantum computation: qubits, quantum states, quantum gates, measurement, density matrices, trace, partial trace the no-cloning theorem.No background in quantum mechanics or quantum information processing will be assumed. The theory is developed from the basics, assuming the postulates of quantum mechanics. Pre-requisite A course on classical error-correcting codes ( E2 205 or equivalent)Ĭourse Outline This course is intended to serve as an entry into the field of quantum error-correction. Grading The course grade will be based on the following: 25% for homework assignments, 25% for the midterm, and 50% for a course project that will involve a written report and presentation.
That will be made available through the MS Teams site for this course.Įxams There will be one midterm exam, to be held on Thursday, March 9, during class hours. These may sometimes be supplemented with additional problems Homework Assignments Homework assignments consist primarily of exercises givenĭuring lectures. The first lecture will be on Tuesday, January 3. Tuesdays and Thursdays, 5:30-7:00pm (tentative)


 0 kommentar(er)
0 kommentar(er)
